5
WELFARE
Annual income twenty pounds, annual expenditure nineteen pounds nineteen
and six, result happiness. Annual income twenty pounds, annual expenditure twenty
pounds ought and six, result misery.
--Charles Dickens
It is impossible to show that a feasible nonmarket system at least approaches the productivity of the market unless a rather well developed theoretical model of the nonmarket system is available.
-Allen Buchanan
In this chapter we present a formal model of participatory planning to compare
its convergence and efficiency properties with those of formal models of market
and centrally planned economics. We show that:
1. A formal model of participatory planning can attain optimal outcomes under less restrictive assumptions than necessary for formal models of market and centrally planned economies.
2. While there is good reason to believe that real world versions of market and centrally planned models will perform less efficiently than analyses of their formal models predict, there is good reason to believe that real world versions of participatory planning would perform better than their formal model predicts. Realistic markets and central planning aggravate their formal model's failures. Realistic participatory planning reduces its formal model's failures.
Preliminary Insights
Differences Between PE and FMPE
Interpersonal Empathy
Interpersonal empathy exists if my level of fulfillment depends in part on other's fulfillment, for example, if I feel better when others feel better. We believe this kind of interpersonal empathy existsmy welfare varies directly with other people's welfare--even under adverse circumstances and can become an important factor under favorable circumstances. Since PE is designed to promote this sentiment, our description of PE incorporated features based on interpersonal empathy. But we do not assume interpersonal empathy in FMPE and do not include features such as the exchange of qualitative information about concrete situations discussed in FMPE. We exclude these features both because they arc difficult to model formally, and, more important, so we can see what conclusions can be derived even if actors in the economy had no interpersonal empathy. Specifically, we want to demonstrate that convergence and optimality results for participatory planning do not depend on the existence of empathy among participants.
Global Motivations
A second difference arises because in PE each consumer knows that she or he is also a worker. The quality of someone's work life depends on the overall demand for goods and services, which depends on per capita consumption, which is implicit in each individual's consumption proposal. Thus, global interactions in PE provide additional motives for its citizens to make socially responsible work and consumption proposals. In PE, each actor considers total welfare which includes preference fulfillment and preference development effects resulting from both her or his consumption and production activities. Moreover, the participatory actor understands that under equitable conditions it is in every individual's interest to maximize over all social well being given the productive skills, equipment, and resources available. This is why PE's actors will know, for example, that in the (not so) long run they have more to gain from investments that greatly improve the quality of work life in other people's workplaces than from investments that only slightly improve the quality of work life in their own workplace. If investment raises work quality locally, PE eventually distributes the benefits to all.
In FMPE, however, we assume people maximize as consumers and workers independently and can "capture" local improvements within the leeway permitted by specific constraints. Again, the reasons are ease of modeling and demonstrating that particular conclusions do not rely on assuming that people have what some may regard as "an advanced state of consciousness."
Levels of Involvement
FMPE contains only neighborhood and workers' councils so that none of the federations of consumers' councils or of regional and industry workers' councils are modeled explicitly in FMPE. As we explained earlier, organizing consumption in nested federations helps create an "incentive compatible" system of expressing preferences for public goods. Similarly, regional federations of workers' councils help to efficiently resolve "production externalities," and industry federations can deal with economies of scale. Since the reason for creating FMPE is to test the efficiency properties of PE, one might question deleting federations from the formal model. The answer is that they are not included explicitly because they do not have to be, as we will see. But this is not to say they are not critical features for ensuring efficiency in PE.
Facilitation Procedures
In PE we allowed for behavioral rules that would limit the range of responses of actors and facilitate price adjustments as the iterations proceed. In contrast, FMPE assumes nondiscretionary, arbitrary percentage adjustments. Although the discretionary rules in PE are themselves somewhat arbitrary and chosen only to hasten convergence with minimal disruption of efficiency and equity, their presence nonetheless constitutes a difference we need to bear in mind.
Available Information
Arguably the most dramatic difference between FMPE and PE is the widespread use of qualitative information in the latter. The fact that consumers and producers in PE have access to detailed descriptions of how people work and consume, and the effects of each, allows actors' economic involvements to be more "human" than in any system entirely dependent on quantitative prices. Again, ease of modeling and a desire to test if particular conclusions hinge on this information dictate deleting qualitative information from FMPE.
Summary of Differences
In sum:
1. FMPE does not account for interpersonal empathy.
2. FMPE does not account for "global" awareness.
3. FMPE does not explicitly define federations of consumption or production councils, although FMPE can be interpreted so as to incorporate their effects.
4. FMPE contains no special convergence hastening procedures.5. FMPE has less robust informational features.
At various points in this chapter, we will return to these differences to assess whether results for FMPE should also apply to PE.
Modeling Consumption
In PE consumers councils make proposals that maximize their members' well-being
and development without presuming preferential treatment for themselves, or,
in other words, subject to the constraint that all other consumers have equal
opportunity to do so as well.1
More specifically, each consumers' council maximizes its own members' well-being and development subject to the constraint that it should not presume to use more of society's scarce productive resources and sometimes debilitating labor efforts per member than, any other council, unless special dispensation is given.
To model consumption behavior in FMPE we remember, therefore, that consumers in local PE councils collectively develop a request for goods, taking into account effects on their immediate well being and on their developmental capacities, which together constitute their total economic well being. Let W(h) represent the total economic well being of consumers' council CC(h) as evidenced by their collective requests. Let U(h) represent the immediate well being of consumers' council CC(h). Let C(h) be the vector of the human characteristics of CC(h)'s members which define the abilities of the members of CC(h) to garner well-being from engaging in various consumption activities. Let the changes in human characteristics of the people in council CC(h) be DELTAC(h), and note that the motivation for desiring a particular DELTAC(h) will be to arrive at I more favorable C(h) from which to derive well-being in the future. 2
Then the welfare that a consumers' council seeks to maximize in its consumption
planning efforts is:

Now let:
x be a vector of production activity levels
A be the input matrix of produced goods for those activities
(intermediate inputs)
K be the capital input coefficient matrix (machines and plant
capacity that must be on hand to produce outputs)
k* be the vector of available capital goods
R be the input matrix of nonproducible, scarce resources
r* be the vector of available, nonproducible, scarce resources
L be the direct labor input matrix of different kinds of labor
I* be the vector of available labor inputs
v be a (row) vector of relative social values of produced goods
So [A,K,R,L] constitute the technical relations of production in the economy and include multiple techniques. But we assume for now that each activity produces a single output so we can identify industries by the goods they produce. An elementary result is that if Pk is the vector of shadow prices for scarce capital goods, Pr is the vector of shadow prices for scarce nonproductive resources, and Pl is the vector of shadow prices for different types of labor, the vector (Pk,Pr,Pl) is the solution to the dual of the primal programming problem for the entire economy.
The primal problem would be:


And the dual problem would be:

Where the dual constraint can also be written

which simply says that shadow prices must be such that the value of all the
resources and labor used both directly and indirectly in any activity engaged
in, plus the value of all the capital goods committed to the activity both directly
and indirectly, must be at least as great as the social benefit from the activity.
Now, suppose ![]() is a vector of particular quantities of different kinds of produced goods requested
by council CC(h). Then
is a vector of particular quantities of different kinds of produced goods requested
by council CC(h). Then

would be the quantifiable
social cost of consumption proposal ![]()
We should note that if the shadow prices for labor were derived from the solution
to the dual programming problem, as stated, then while they would reflect the
different productivities of different kinds of work-the result of labor supplies,
technological possibilities, and preferences for goods-they would not reflect
differential desirabilities from the workers' point of view. In which case the
above equation would not be a completely accurate indicator of the social cost
of a consumption proposal. However, the shadow prices for labor generated by
the iterative planning procedure we describe below for FMPE do include this
component. And since the shadow prices we use are those derived from the FMPE
procedure defined below, not the shadow prices from the dual programmming problem
discussed in this heuristic explanation of the problems faced by consumer and
producer actors in our economy, the estimates of social costs we calculate in
FMPE do reflect the differential desirabilities of different categories of work.
This heuristic presentation is sufficient to illustrate the logic of consumption. Consumers in CC(h) propose bundles they like considering both the preference fulfillment effect and the changes they expect their consumption activity to produce. That is, consumers seek to maximize their own total economic well-being, W(h), where
![]()
![]()
is greater than, less than, or equal to zero, where
![]() is the
average consumption bundle requested by the H consumption councils in the economy
which, for convenience, we assume have the same number of members.
is the
average consumption bundle requested by the H consumption councils in the economy
which, for convenience, we assume have the same number of members.

Differences from Participatory Consumption
As we noted earlier, this model deviates from participatory consumption in some respects.
1. To correspond to the consumption side of a real participatory economy, the model would have to explicitly include individuals.
The absence of individuals is a minor difference that can be handled in two ways. We could confine each unit in FMPE to one member. Or, more realistically, we can note that if the council FMPE model yields desirable results, nothing precludes the individuals in each unit from dispersing the group's "bounty" among themselves efficiently and fairly.
2. There is a difference regarding consumers' presumed goals. In FMPE consumers try to maximize their own well-being and development without including as one of their goals the well-being of people in other units.
To be sure, FMPE's consumption constraint ensures that equity is preserved, but it incorporates no institutional or motivational sensitivity to how the well-being of some depends on the well-being of others. Actors in PE, on the other hand, are capable of expressing and developing solidarity. This difference, however, does not mean PE will not perform as well as FMPE.
While a significant difference, again this does not diminish the likelihood that PE will perform as well as FMPE.3. FMPE includes no mechanism for communication or use of qualitative data.
4. The absence of explicitly defined federations of consumers in FMPE appears to be a significant difference from PE that would certainly impact on the efficiency properties of FMPE.
However, FMPE is sufficiently abstract so that if and when it suits our purposes we can interpret any of the abstract consumers' councils in FMPE as a ward, city, state, or national federation of consumers' councils.
In other words, CC(h) may be a neighborhood consumer council, but CC(w) can be interpreted as a ward council, CC(s) as a state federation, and CC(n) as the national federation. So as long as it is clear that consumption federations participate as actors in the iterative planning process just as neighborhood consumers' councils do, that well-being functions for federations represent the average preferences for public goods of all members of a federation, and that consumers are "charged" their proportionate share of federation requests, FMPE is sufficient to "test" PE with regard to efficiency even under assumptions of public goods and external effects, as will become clear.Modeling Production
Traditional economic theory treats work units as places where physical materials are transformed via an unspecified process employing human labor. The only concern is whether the transformation occurs efficiently. Could inputs be used to generate more outputs? Could we achieve the same physical transformations with less or less disagreeable labor?
In contrast, we view a workers' council, WC(j), as another center of human activity that has both short-run and long-run human consequences for those involved as well as repercussions for others. Moreover, the workers in PE have specific information permitting them to consider the well-being of other workers and consumers. And PE's participatory workers know they are consumers and that their consumption depends on total production, which in turn depends on their work proposals. But while these features of production in PE are not included in FMPE, we do model workers' councils in FMPE as centers of human activity that affect the immediate well-being and future characteristics of those participating as well as the prospects of others in the economy.
Constraints
In PE, actors know that job complexes will be balanced across workplaces and that consumption is tied to work effort. This makes it advisable for workers to make proposals that maximize overall social welfare since under equitable arrangements this also maximizes the benefits to each. But in FMPE we do not assume workers necessarily understand this. Instead, we assume workers in each council, WC(j) make proposals to maximize their own well-being and development from work, W(j) = W(U(j), DC(j)) But in attempting to maximize their own well being workers' councils will be subject to some constraints. For example:
1. We do not want some workers working short hours, or at a leisurely pace, or in luxury conditions while others work long hours, or at breakneck speed, or in dangerous conditions.
2. We do not want some workers' experiences to be significantly more "empowering" than others.3. We do not want some workpl aces using excessive quantities of scarce resources, valuable machinery, and produced inputs to generate very little or unwanted output.
4. We do not want people trained as mining engineers working in road construction while civil engineers work in mines.
Constraints 3 and 4 are typical of the traditional economic demand for productive efficiency and are explicitly addressed in our formal model, but we choose not to address constraints 1 and 2 this way. We want workers to make their own work lives as fulfilling and empowering as possible without presuming a more privileged position for themselves. We could require the planning procedure to equalize work conditions in all councils, so that in addition to each worker within a council having a situation comparable to that of all others in the same council, each council would have the same average conditions as every other. We reject this approach because it destroys diversity, is unnecessarily inefficient, and tends to reduce the quality of worklife to the lowest common denominator. Instead we allow councils to develop very different work conditions from one another but require that workers in councils with nonaverage work conditions balance their work time among two or more councils so all workers enjoy comparable effects.
In other words, in FMPE we assume that different groups of workers participate in the planning process by attempting to maximize the quality of work life in their primary workers' council subject only to constraints that address points 3 and 4. After the plan is settled workers split time between units so as to address points I and 2. So the planning procedure in FMPE is responsible for addressing inefficiencies (3 and 4), while inequities and disparate empowerment (1 and 2) are addressed in a post-planning shuffling of assignments and schedules organized by economywide Job Complex Committees.
Therefore, in FMPE we treat workers' councils in a way analogous to the way we treated consumers' councils. Each workers' council tries to maximize the total well-being of its members' work experience subject to the constraint that it be as useful to the rest of society in any work that it performs as any other equally endowed workers' council.
Different Productive Endowments
Specifying "equally endowed" is key to modeling production in FMPE. A particular workers' council is defined by its members' training and skills and by the physical productive assets (plant and equipment) at its disposal. That is, all workers' councils have unique productive capabilities, but not all workers' councils are created equal. Some have greater per capita capabilities, some less. We do not want some wellendowed workers' councils taking advantage of other less wellendowed ones by making proposals that for lesser endowed councils would represent considerable work efforts and sacrifices, but for a council with a large endowment represents insufficient efforts.
But how do we define and measure "equally endowed"? And even if there is an effective way to classify workers' councils (WCs) into different groups that are "equally endowed" with respect to productive capabilities, how can we measure the extent to which one group of "equally endowed" WCs is more or less capable than another? Ultimately all WCs'proposals must be measured against one another in order for participants in the planning procedure to judge which proposals represent acceptable efforts and merit approval, and which do not.
As with consumption, indicative prices play a critical role comparing the productive endowments of different WCs. If, as in the case of consumers' councils (CCs), we assume a vector of known indicative prices, we can measure productive capabilities of any WC as well as the social costs of any intermediate inputs it requests and the social usefulness of the outputs it proposes to deliver.
In thinking about how to define FMPE, it is clear that a "circulating capital" model is inappropriate since this approach would fail to clarify what distinguishes one WC from another. In a circulating capital approach, differential productive endowments disappear as no unit is characterized by anything other than an identifying number. Likewise, in a Debreuvian model the different production units are distinguished by different production possibility sets, but not because the firms in the models each begin with particular machinery or with personnel having particular skills and knowledge. In Debreuvian models each production center has a unique book of productive blueprints-hence the differential profits that accrue to different firms in Debreuvian competitive solutions. But certainly the first thing a participatory economy would do is publish all its books of blueprints! It follows that we cannot usefully employ Debreuvian assumptions. In Sraffian or Von Neuman models industries are defined but all firms in an industry are presumed to have access to the same technology, and to use the same technology to produce their product(s). Clearly this will not help us represent WCs as units with distinct human/physical productive capabilities.
In sum, we want to recognize the existence of particular people with particular historical work experiences, skills, and capabilities. We cannot quantify all aspects of what makes workers' councils unique. For example, the social relations established by having worked together in the past cannot be captured in FMPE. But we can account for scarce skills and training and for productive characteristics of plant and equipment of particular vintages.
Workers' Councils
What will make WCs distinct is the combination of human and physical assets they begin with. These consist of the productive characteristics of the members of the WC, and the plant and machines the WC has when planning begins. With this in mind, we are ready to define the production side of FMPE.
Let lj be the vector of the number of units of each category of labor present in the initial membership of WC(j) where
![]()
Let kj be the vector of initial capital stocks (plant and equipment) that characterize WC(j) where

And let ![]() be the
vector of the number of units of each category of capital good WC(j) proposes
to add to or release from its initial capital stock, and
be the
vector of the number of units of each category of capital good WC(j) proposes
to add to or release from its initial capital stock, and![]() be the vector of the number of units of each category of labor WC(j)
proposes to add or release. Then, each WC(j) will initially be "charged" for
its productive resources an amount
be the vector of the number of units of each category of labor WC(j)
proposes to add or release. Then, each WC(j) will initially be "charged" for
its productive resources an amount
![]()
which defines the extent to which socially useful outputs can be reasonably
expected. If the WC(j) is willing to be charged for more productive labor or
wishes to be charged for less, it can submit as part of its proposal at any
iteration![]() >
0 (demanding more of the ith labor type) or
>
0 (demanding more of the ith labor type) or ![]() < 0 (releasing some of the ith labor type). Similarly, if the WC(j) wants
to request more or release some of its previous capital stock, it can submit
as part of its proposal at any iteration
< 0 (releasing some of the ith labor type). Similarly, if the WC(j) wants
to request more or release some of its previous capital stock, it can submit
as part of its proposal at any iteration ![]() >
0 (demanding more of capital type i), or
>
0 (demanding more of capital type i), or ![]() < 0 (releasing some of capital type i). Note that the assumption that
no new capital or labor types become available to the economy as a whole in
the period means that
< 0 (releasing some of capital type i). Note that the assumption that
no new capital or labor types become available to the economy as a whole in
the period means that
![]()
So, if we let ![]() be WC(j)'s vector of proposed inputs of produced goods a
be WC(j)'s vector of proposed inputs of produced goods a ![]() be
WC(j)'s vector of proposed inputs of resources, it
be
WC(j)'s vector of proposed inputs of resources, it
follows that
![]()
represents the social value of the inputs WC(j) is requesting in its proposal,
plus the social value of its productive endowment, corected for proposed additions
and releases. If ![]() is the vector of outputs WC(j) proposes to supply, then
is the vector of outputs WC(j) proposes to supply, then ![]() is the social value of those outputs, and as long as
is the social value of those outputs, and as long as
![]()
WC(j)'s proposal will not be what we might call "socially abusive." (Recall that the dual of the societal programming problem finds shadow prices such that

with strict equality for any activity that forms part of the optimal solution.) In formal models of traditional economies, production units are assumed to maximize
![]()
subject to the constraint of known technology. In our model, however, WCs try to maximize their member's well-being-that is, their fulfillment and development-subject to the above constraint. We let U(j) represent the members' immediate fulfillment and DC(j) represent their development so that W(j) = W(U(j),DC(j)) represents their wellbeing function.
Differences from Participatory Production
Once again, our model approximates but does not fully represent participatory
production.
1. There is a difference in what workers take into account in making their choices. In FMPE, producers try to maximize their own wellbeing assuming that it does not depend on others.
Again, constraints ensure that equity is not violated, but no empathy is assumed,
and no solidarity enhancing features are incorporated.
2. FMPE includes no mechanism for the use of qualitative data.
Again, as we will see, this difference proves not to diminish the likelihood that PE will perform as well as FMPE.
3. No federations of workers' councils are explicitly defined in FMPE.
Again, we will discover that by interpreting some WC(j)s as geographically based federations and some as industry federations, production externalities and economies of scale can be accounted for in FMPE in ways that reflect how they would be treated in PE.
Formal Summary of Councils' Goals
At this point we can summarize the problems facing consumers'
and workers' councils in FMPE. The problem for a typical consumer council, CC(h),
is

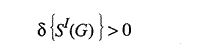
where ![]() is the average consumption bundle requested so that this expression represents
an equitable budget constraint on consumer in FMPE.
is the average consumption bundle requested so that this expression represents
an equitable budget constraint on consumer in FMPE.
The problem facing a typical workers' council, WC(j) is:
![]()

which says workers are free to maximize the quality of work life as long as the social value of their contribution to output exceeds the social costs they incurred in producing it. Now if I the well-being functions of consumers' councils had all the convexity properties traditionally assumed in formal analyses for individual consumer's exogenous preferences, the consumption constraint above, read as an equality, would be a separating hyperplane between CC(h)'s "at least as preferred consumption set" and the "socially nonabusive" (not too greedy) consumption set. And if the well-being functions of workers' councils had similar convexity properties and workers' council's production possibility sets had the same convexity properties usually assumed, then the production constraint above, read as an equality, would be a separating hyperplane between WC(j)'s "at least as preferred production set" and its "socially nonabusive" (not too lazy) production set. Under these assumptions, objectives of CCs and WCs could be integrated by the planning procedure presented below to yield familiar convergence and optimality properties. That is, the formal participatory model, FMPE, whose nonmarket, noncentrally planned procedures we describe formally below, proves at least as viable and desirable as familiar market and central planning models.
It is worth noting the similarity between the planning problems faced by CCs and WCs in FMPE. This formal equivalence reflects our underlying insight that work and consumption are each instances of social economic activity that always affect the well-being of those immediately involved as well as the prospects of others. So, in the case of both consumption and production, the choice problem, as we conceive it, is for groups of people to pursue their own well-being subject to the constraint of social responsibility, which in our view translates into granting others equal means to achieve their goals as they see them.
Allocation
We now add a Planning Bureau, PB, that accumulates no information and recalls no information. from previous iterations. As a matter of fact, since the PB's functions in FMPE can be described as a mechanical algorithm, all its functions could be automated. And we are ready to give a formal description of FMPE's decentralized planning procedure.
The actors in the social, iterative, planning process are consumers' councils CC(h)s, workers' councils, WC(j)s and the planning bureau, PB. In total, FMPE is a variant of a well-known price-guided procedure originally developed by Oscar Lange, Kenneth Arrow, and Leonid Hurwicz.
1. Each CC(h) makes an arbitrary, initial proposal ,
Since FMPE consumption proposals may be arbitrary, realistic PE
consumption proposals made in light of last year's plan, indica tive prices,
and expected or desired growth should require fewer iterations to converge.
Again, the fact that FMPE allows the initial proposal to be arbitrary means PE proposals based on last year's indicative prices and workers' knowledge of consumer and worker desires expressed in early iterations last year should converge in fewer iterations.2. Each WC(j) makes an arbitrary initial proposal,
Usually this is taken to be3. The PB quotes an arbitrary price vector
4. Each CC(h) changes its request for good i according to the rule:
![]()
![]() the
total net production vector proposed by all WC in step 2:
the
total net production vector proposed by all WC in step 2:
![]()
H = number of consumers councils
and a = an adjustment coefficient between 0 and 1:
0 < a < 1
This merely says:
a. If a CC estimates that the increase in its well-being from obtaining a first unit of good i is less than the value of what it will be "charged" by other councils for the resources producing good i requires, it should not ask for any good i.
b. If a CC estimates that the change in its well-being from obtaining an additional unit of good i is greater (less) than the value of what it will be "charged" by other councils for the resources producing good i requires, the CC should increase (decrease) its request for good i by some fraction of the discrepancy.
It should be clear that this implies that consumers' councils will change their requests whenever the difference between social benefits and social costs would be increased by doing so. If CCs in FMPE propose no changes it is because they have reached local optima, having satisfied the necessary and sufficient Kuhn-Tucker conditions. The difference in PE is only that estimates of social cost are based not only on current indicative prices-which is the only thing considered in the formal model-but qualitative information including graphic descriptions of the human consequences of producing and consuming different goods. Again, the additional information available in PE can only improve the quality of estimates of true social costs and benefits. It is also the case that adjustments in PE are not confined to an arbitrary proportionality factor that is independent of the degree of excess demand and supply.
5. Each WC changes its proposal by increasing, decreasing, or leaving unchanged
the ![]() and implicitly
and implicitly
![]() according to
the following rules.
according to
the following rules.
![]()

where b = an adjustment coefficient between zero and 1: 0 < b < 1
Remember that other councils primarily judge workers' councils' proposals with
an eye to the relation between the social benefits of heir outputs and the social
costs of their inputs. These rules together express the idea that if changing
the input/output mix increases net social benefits a workers' council will implement
the change. Or if he increased well-being of the workers involved outweighs
any excess of social cost over social benefits to others that accompany he change,
the change will be enacted. But this implies that wheneer producers can adjust
production to generate a positive net social benefit, they will. The fact that
PE provides additional qualitative information beyond indicative prices means
PE's actors will better esstimate social costs and benefits. Likewise, more
flexible adjustment coefficients can facilitate more rapid convergence. Moreover,
PE employs various rules limiting responses of actors during some iterations
The advantage is that these devices can accelerate convergence. The disadvantage
is that they may induce minor inequalities and inefficiencies. The trick, of
course, is to choose regulations whose time savings are more valuable than inequalities
or inefficiencies introduced.
6. The PB changes prices
according to the following rules

where g = an adjustment coefficient between zero
and 1: 0 < g < 1
These rules raise prices for goods in excess demand and lower prices for goods
in excess supply, thereby moving proposals toward a feasible plan. The difference
between PE and FMPE here is that in FMPE price adjustments are always the same
arbitrary percentage of excess demands, whereas PE adjustments can vary to hasten
convergence. We leave discussion of this to chapter 6.
The rest of the planning procedure in FMPE simply repeats steps 1 to 6 until there are no further changes. The proof that this procedure, which, as we indicated, is a variant of a procedure developed by Lange, Arrow, and Hurwicz, 3 will converge to a feasible and optimal plan under the usual convexity assumptions can be found in chapter 4 of The Theory of Economic Planning by G.M. Heal (Amsterdam: North Holland Press, 1973). 4
While it is mathematically equivalent, economically, our procedure differs substantially from the Lange-Affow-Hurwicz procedure discussed by Heal. We have consumers' councils maximizing their wellbeing subject to constraint while Heal stipulates an overall social welfare function. And we have workers' councils maximizing their wellbeing subject to a constraint, whereas Heal stipulates profit maximization. However, these economic differences notwithstanding, the convergence proof Heal outlines for the LangeArrow-Hurwicz procedure applies to our procedure as well.
What our social iterative procedure essentially does is "whittle down" infeasible proposals from both consumers' and workers' councils in two different ways.
1. Unjustifiable consumer "greediness" is reduced by the refusal of other consumers' councils to approve requests that require a greater than per capita use of society's scarce productive resources while unjustifiable worker "laziness" is reduced by refusal of other workers' councils to approve requests that entail less than average work effort. This kind of whittling is expressed in the constraint inequalities for consumers' and workers' councils dictated by equity.
2. At the same time, excess demand for particularly scarce inputs and socially costly outputs are whittled down by raising the indicative prices that units requesting them are "charged" while excess supplies of plentiful outputs and socially inexpensive inputs are reduced by lowering the indicative prices that units requesting them are charged. Wherever possible, these changes in relative prices induce optimizing councils to "shift" their requests rather than "reduce" their requests, so that equity and efficiency are generated simultaneously.
In technical terms, convergence and optimality hinge on the convexity properties of our consumers' and workers' councils' well-being functions and the production possibility sets of producers. But while convexity of production possibility sets is a well known issue, the convexity properties of our council well-being functions are not. Moreover, our consumers' councils differ from the consumers of traditional theory in three important ways. First, our consumers' councils consist of a number of people whose individual preferences presumably differ. Second, some consumers' councils in FMPE are lower level neighborhood councils, but others are higher level federations of councils so that the well-being functions of some councils are really the well-being functions of groups of neighborhoods, wards, or states for public goods. And third, while we could have treated preferences as exogenous in FMPE and recognized only "preference fulfillment effects" of consumption choices, we insisted on allowing for endogenous preferences and recognizing the importance of "preference development effects" as well. We reviewed our reasons for doing this in chapter 1 and developed a rigorous analysis in chapter 6 of Welfare Economics where we also explained why, in our view, the more realistic assumption of endogenous preferences makes traditional assumptions of convexity less plausible.
Our modeling of workers' councils is even further removed from traditional treatments of production. Besides treating group rather than individual preferences for work life and recognizing the preference development as well as preference fulfillment effects of work choices, we have formulated worker choice in the same terms as consumer choice. Since this is unusual, there has been very little previous discussion of what the convexity properties of such preference orderings might be like.
However, in our opinion, for most economists convexity assumptions have long been an assumption of convenience rather than conviction. The reasons for doubting the plausibility of convexity assumptions necessary for proving the existence of equilibria in market economies and convergence to feasible plans in different versions of central planning are compelling and well known. And of course recognizing the endogeneity of preferences adds yet another reason for doubting that reality is conveniently convex. But historically the necessity of ignoring these doubts in order to proceed with formal analyses has been, for the economists concerned, more compelling still. In this vein, we do not see that working through the logic of our economic model under what are admittedly dubious convexity assumptions is any different than doing the same for traditional models of more familiar economic systems. It is a reasonable procedure in treating formal models precisely insofar as it illustrates one important respect in which real systems, like PE, should diverge from formal models, like FMPE, if they are to prove useful. Some of the differences between allocation procedures in PE and FMPE are necessary, that is, because the convexity assumptions required to guarantee convergence for FMPE cannot be expected to hold in the real world setting of PE.
Summary
We have presented a "well-defined" formal model of an economic system quite different from familiar formal models of individualistic consumption and hierarchical production integrated by markets or central planning. FMPE has participatory, social, and equitable properties consistent with our version of PE and we have demonstrated how under the same assumptions necessary to prove coherence and optimality in familiar models, FMPE will also converge to a feasible, Pareto optimal plan. This provides an answer to Allen Buchanan's request for a "well-developed theoretical model of the nonmarket system."
As we explained in chapter 3, if higher level federations of consumers' councils participate in the planning process equally with neighborhood councils-proposing and revising requests for "public goods" appropriate to their level-and if individuals are "charged" their proportionate share of the social costs of those requests, there is no incentive to misrepresent preferences for public goods or to try to "ride for free." Moreover, federations provide realistic settings for implementing further refinements consistent with efficiency along the lines of demand revealing and pivot mechanisms discussed in the theoretical public finance literature. In sum, if we recognize that some CC(h)s in FMPE are higher level federations and assume a gradation of federations that corresponds to gradations in differences between issues of local and central finance, FMPE generates accurate estimates of social benefits for public as well as private goods, and correspondingly optimal plans.
The geographic-based federations of workers' councils permit analogous consideration of production externalities. In the traditional example of downstream producers, the federation of workers' councils along the stream would express the "demand" for clean water for production purposes. There is neither incentive for the federation to exaggerate the benefit of clean water to them-since there is no issue of "compensation" for damages-nor is there incentive to underestimate the negative impact of a pollutant. The polluter may have a short-run incentive to underreport the amount of pollution since the workers' council responsible will be "charged" a negative indicative price times the quantity of pollution released, but this incentive is no different from an incentive to underreport the stock of machinery on hand or skill levels of members, since workers' councils are also held responsible for these productive assets. In both cases, accurate measurements of "inputs" and "outputs" are necessary, but this is true in any economy. The advantage of FMPE is its ability to generate accurate evaluations of external effects assuming that the physical quantities can be reasonably estimated.
About half a century ago, Oscar Lange, Abba Lerner, and Frederick Taylor, responded to an erroneous consensus that public enterprise economies could not operate efficiently by elaborating a model of what they called a "socialist" economy that they argued was capable of yielding Pareto optimal outcomes. While not an end to the "socialist calculation debate," their model served as a powerful challenge to what had become a firmly held "impossibility" conviction among economists regarding the supposed inability of public enterprise systems to yield efficient results. Interestingly, their formal model was derived directly from propositions well known to microeconomists of their day. 5
Our formal model of a participatory economy also relies heavily on work well known to microeconomic theorists. The planning procedure is a variation of a "price guided" procedure well known to those familiar with the literature on iterative planning mechanisms that flourished briefly in the late 1960s and early 1970s. It is true that this literature focused on solving what was considered at that time the principal problem of central planning-how the central planning bureau could gather information about the technical capabilities of different units-while our focus is to adapt iterative procedures so that the different units in the economy can participate directly in forging an equitable, efficient plan without the intervention of any central planning bureau. But as in the case of Lange, Lerner, and Taylor, the techniques we use have been familiar to microeconomic theorists for decades. We hope our formal model will also challenge unwarranted pessimism among economists, namely that there is no alternative allocation mechanism to markets or central planning. Decentralized planning in which groups of workers and consumers participate directly in arranging their own economic endeavors is not only feasible, it is highly desirable.
Formal Models Versus Reality
In our view, economic theorists have often engaged in a kind of deception we do not wish to continue. Based on a legitimate appeal to the practical necessity of abstracting from countless intricacies in order to focus on a relatively few key relations for the purpose of discovering their non-intuitive consequences, economists have constructed abstract, formal models of different economic systems. The most familiar is the "ideal" model of a competitive, private enterprise, market economy known to all who have taken a course in microeconomic theory as "perfectly competitive capitalism." But similar "ideal" models of centrally planned public enterprise economies and variants of public enterprise market economies have been constructed as well. The deception arises not in the derivations of conclusions from stipulated assumptions, but in the examination of the realism of the assumptions and the consequent need to question the realism of the conclusions derived from those assumptions. In particular, conclusions about the desirability of different economic institutions and systems as well as policy recommendations that stem from conclusions drawn from formal models without attempting to estimate the effects of predictable discrepancies between reality and the model's assumptions can be very deceptive. Here we summarize the most important differences between the assumptions of FMPE and the reality of PE, and between the procedures of FMPE and the procedures of PE in order to assess the plausibility and usefulness of the conclusions derived from FMPE for our assessment of PE itself. But first we summarize our assessment of traditional alternatives in the same light.
Ideal Markets Versus Reality
If real markets equilibrate instantaneously, if real market structures are competitive, if real buyers and sellers have perfect knowledge of all prices (past and future), and if there are really no externalities or public goods, then and only then would formal models allow us to conclude that real market systems will yield prices that accurately reflect true social benefits and costs and Pareto optimal allocations of resources and goods. But if any of these assumptions are not met in real systems, real market prices will mis-specify real social costs and benefits and real market allocations will be socially inefficient. It is not our purpose to review the vast literature on market disequilibria, market imperfections, and market failures. All these are important, but in our opinion the pervasiveness of external effects is alone sufficient to render market failure ubiquitous, and consequently, to render market misallocation the rule, not the exception, as we have explained at length in Welfare Economics. But the point is this: (1) To the extent the assumptions of formal models are unwarranted, the model's conclusions about efficient allocations of resources are unwarranted as well. (2) There are ample reasons to doubt the plausibility of some if not all the assumptions for market models. Our two contributions to the skeptical literature on markets have been to argue that external effects are pervasive, and that the bias against providing goods with greater than average positive external effects inherent in any conceivable real world market economy, in context of endogenous preferences, will lead to ever increasing inefficiencies. 6
One approach for dealing with public goods is the GrovesLedyard demand-revealing mechanism in which individuals report to the government their marginal willingness to pay for all quantities of every public good. I report how much I am willing to pay for one missile, two missiles .... one road, two roads .... and so on. The government supplies a level of each public good such that the marginal social cost of production equals the sum of the marginal willingness to pay of all consumers. Each individual is then taxed according to her or his proportionate share of the total expenditure minus the reported consumer surplus of all others. I pay my share of the cost of all public goods minus the difference between what all other citizens have said they would be willing to pay for the amounts provided and their proportionate share, and so- does everyone else. The critical idea is that my tax bill is effectively independent of what I myself report to the government regarding my taste for public goods. I cannot lower my bill by reporting inaccurate information The only thing I can do by giving information to the government is influence the amount of each public good provided, and I can do this best by accurately reporting my preferences for public goods.
Theoretically, it works. But note that each individual has to report to the government her or his desires for every possible quantity of every public good. It is not simply that we have to say how much we think should be delivered. We must say how much we would like the delivery of each possible amount of every public good. Imagine the information apparatus necessary to communicate all this. It is an information flow comparable with what participatory economies require for the entire economy because, while dealing with fewer goods, it addresses a much broader scope of quantities. Now imagine incorporating this entire apparatus on top of a market system. The "public goods sector" would approach an information capability sufficient to allow collective self-management along the lines of PE, but the "superior" system would be used for only a subsector of the economy. Trying to introduce the Groves-Ledyard corrective mechanism would ultimately subvert the market system.
Ideal Central Planning Versus Reality
A similar argument applies to central planning. If real central planners knew the technical capabilities of all production units (or if there were incentive compatible procedures to induce real units to reveal their true capabilities to central authorities), if real planners knew all primary resources availabilities, if real planners knew the relative social benefits of all goods, if real planners could count on their orders being faithfully executed, if real jobs were assigned and goods distributed efficiently, and if nobody valued having decision making input in proportion to the degree they were affected by the outcome, then and only then would formal models of centrally planned economies allow us to predict Pareto optimal outcomes for real centrally planned economies. While we find usual "Western" assessments of the information and incentive problems of central planning more skeptical than warranted-particularly in light of an asymmetrical unwillingness to examine the assumptions behind market models with a similarly critical eye-we nonetheless concur that discrepancies between realistic central planning and formal models of central planning make it naive to translate conclusions from the formal models to the real world.
In realistic systems, where a coordinator class substitutes its own welfare function for that of ordinary citizens, obviously central planning does not maximize social welfare. Yet, even if this could be avoided, there is no way to eliminate the bias against selfmanaged work opportunities inherent in even the most democratic central planning without eliminating the basic hierarchical decision making relation defining central planning. But once we entertain the idea of doing this, we would have ' to institute new means of information dissemination that would move us toward participatory economics. On the other hand, if we maintain central planning's inherent bias against self-management, then central planning would inevitably involve a spiral of authoritarianism/passivity and associated snowballing divergence from Pareto optimality, as proved in Welfare Economics.
FMPE Versus PE
Besides "practical" problems of disequilibria and market imperfections, the principal discrepancy between formal models of market economies and real world market systems is the pervasiveness of external effects in the latter. Besides "practical" information and incentive problems, the principal discrepancy between formal models of centrally planned economies and real centrally planned economies is that it does matter to real people who decides what they will do. Not surprisingly, the price of ignoring these discrepancies is failure to understand the fundamental deficiencies as well as practical problems of market and centrally planned allocations. That is, accepting conclusions from formal models that fail to recognize that human beings are distinguished by their ability and desire to make choices about how to conduct their lives (models of central planning), and formal models that fail to recognize that human beings are a social species, and therefore that our economic activities are characterized by varying degrees of external effects (models of market economies), risks seriously misestimating the usefulness of these allocative mechanisms to real human beings.
The question now is whether conclusions derived from FMPE could be deceiving as well. First, FMPE was specifically designed with what we believe are the fundamental inadequacies of markets and central planning in mind. By including "nested" federations of consumers' and workers' councils among our actors in the planning system, we have accounted for complex sociality in human economic endeavors at the ground level of our economy rather than pretending sociality is a rare and unimportant occurrence or compensating for it as an afterthought. And by having workers' and consumers' councils propose and revise their own activities we have guaranteed "grass-roots" participation. But this is not to say that FMPE is completely sensitive to human sociality and self-management.
FMPE includes federations of councils only via the theoretical artifice of interpreting CC(h)s conveniently. While such artificeslike the Debruvian artifice of treating time by interpreting the same good in different time periods as different goods-are useful, they are also often unsatisfactory in important respects. In our case there is a lot more to the integration of federations into the real planning process in PE than is indicated by the formal treatment in FMPE. FMPE also operates entirely at the level of workers' and consumers' collectives, which leaves individual participation out of the formal model. However, PE includes individual worker proposals and permits living units and their members to propose their own individual consumption activities.
More significantly, in FMPE actors have no qualitative information concerning one another and are not assumed to behave out of empathetic feelings. The flow of qualitative information we described in PE is deleted in FMPE simply because it is difficult to formalize. On the other hand, we assume actors in FMPE are motivated by individual self-interest and never by empathy for an important reason. The reason is not that we believe human beings are incapable of acting out of concern for others, since we believe they do so under propitious circumstances and frequently even in situations that discourage such behavior. Nor is the reason that we attach little importance to solidarity in evaluating economic affairs. Quite the contrary, we consider promoting solidarity one of the chief virtues an economic system can claim. Instead, we assume actors in FMPE operate entirely out of selfinterest in order to answer legitimate objections from skeptics.
That is, if the kind of economy we espouse could only function efficiently if actors made choices based on concern for one another, it would only be of value for people who have already achieved a high degree of mutual concern. But we claim to have designed an economy that promotes solidarity by overcoming mistrust and antagonisms based on real historical experiences of exploitation and oppression by building a legacy of equitable, mutually beneficial institutions. That is, we claim individual self-interest coincides with the social interest in PE, and PE's institutions lead people to take the interests of others as seriously as they take their own when making decisions.
So, we reiterate, the conclusions of FMPE are not based on any assumption of empathetic behavior on actors' parts. In FMPE actors are assumed to be the same "homo-economi" as in traditional models-doing the best they can for themselves under the circumstances in which they find themselves. In PE there is additional qualitative information provided that promotes development of solidarity and in PE there are opportunities for granting exceptions to rules concerning effort and consumption based on empathy for others' needs. Thus, to the extent that real human behavior in PE deviates from individual self-interest and incorporates a degree of solidarity, PE will function better than conclusions from FMPE predict.
In sum, while no real economy will ever achieve perfect estimates of social costs and benefits of all production and consumption choices, and consequently no real economy will ever reach a Pareto optimum, we do not believe PE would be characterized by the systematic biases inherent in traditional economic systems. Participatory economies would not be biased against activities with greater than average positive "external" effects and would not make it more difficult for people to engage in self-managed work than necessitated by real technological and resource constraints. Still, if imperfections are inevitable, and if endogenous preferences inevitably magnify initial imperfections over time as we have argued in Welfare Economics, will not PE eventually careen down some increasingly nonoptimal trajectory, even if it is a different one than those traveled by centrally planned and market economies? To the extent that people are capable of "purposeful preference molding" or "informed self-development," biases in economies will become magnified over time and systematic nonoptimalities will snowball, as we spelled out in Welfare Economics. But this does not imply that pursuit of economic efficiency is hopeless. The key is that systematic biases will lead to snowballing divergences from optimal allocations. However, if biases are random in the sense that a kind of activity that is "overpriced" one period is just as likely to be "underpriced" as "overpriced" the next, there is no way individuals can adjust to benefit and thereby produce the socially counterproductive effect of aggravating the bias further. So if FMPE contains no systematic biases, then inevitable misestimations in PE need not yield a spiral of increasing divergence from optimality. Moreover, the generation of developmental effects of economic choices that would be the basis of many exceptions to formal rules in FMPE granted in PE would provide an environment sensitive to detecting and correcting deleterious effects of endogenous preference development.Conclusion
In. the context of pervasive externalities and desires for selfmanagement we have shown that: (1) realistic market and centrally planned economies will do far worse than their unrealistic formal models. Far from achieving optimality, once crucial unrealistic assumptions are recognized as assumptions of convenience, each of these familiar systems are seen to contain a systematic bias. Moreover, we have explained why in the context of endogenous preferences systematic biases inevitably lead to a snowballing divergence from Pareto optimal allocations and therefore to increasing degradation of human possibilities. We have also explained why we believe correctives require interactions that would be impossible and/or unstable in these flawed institutional contexts. On the other hand, we have shown that (2) a formal model of a participatory economy, FMPE, in which actors are assumed to behave only in accord with individual self-interest, will achieve Pareto optimality even in the context of external effects and desires for self-management. Moreover, we have argued that realistic versions of participatory economies, PE, should do still better than FMPE. That is, divergences between formal models and real world versions of participatory economies are likely to be self-correcting, whereas just the opposite is the case for formal models and real world versions of market and centrally planned economies. In sum:
1. In the traditional world of abstract formal models, participatory economies deserve to be considered an equally viable alternative to perfectly competitive capitalist and coordinator market and centrally planned economies.2. Formal models of participatory economies achieve Pareto optimality under far less restrictive and more realistic assumptions than formal models of market and centrally planned economies.
3. Realistic capitalist and coordinator economies differ from their formal representations in ways that magnify their failings, while realistic participatory economies differ from their formal representation in ways that enhance their capacity to attain desirable results in fewer steps and at reduced cost.
1. We assume for convenience equal work efforts and no borrowing or lending. Any divergences would be accounted for as explained in chapter 3.
2. This unconventional treatment of preference fulfillment and development as components of total economic well being is further elaborated in chapter 6 of Welfare Economics.
3. K. Arrow, L. Hurwicz,
and H. Uzawa, Studies in Linear and Nonlinear
Programming (Stanford:
Stanford University Press, 1958).
4. The proof hinges
on stability properties of gradient procedures for finding
saddle points which
are in I LaSalle and S. Lefschetz, "Stability in Liapunov's direct method, with
applications," Volume 4: Mathematics in Science and Engineering (New
York: Academic Press, 1961). An extension of the stability theorem to cover
discontinuities in the rate of change of variables when boundaries are reached
and when non-negativity constraints become binding can be found in C. Henry,
"Differential equations with dicontinuous right-hand sides in mathematical economics,"
Journal of Economic Theory 4, no. 3 (1972).
5. For an accessible modem rendition of their work see Oscar Lange and Frederick Taylor, On the Economic Theory of Socialism (New York: Monthly Review Press, 1964).
6. See chapter 7 in Welfare Economics.
